Math educators are on a never ending quest to help students improve their problem solving skills. To succeed on this quest, they must choose between a variety of tools and it can be challenging to determine which are better. So, I wanted to share my take on two main categories I see:
- problem solving tools designed for complicated situations
- problem solving tools designed for complex situations
If you haven’t read my blog post on whether Depth of Knowledge is complex or complicated, you may be wondering about the differences between complicated and complex. I previously used both words interchangeably when I wanted to say “difficult” but sound fancy. Now I see significant differences between them and understanding what those are will make this conversation much easier.
To make this clearer, think about the differences between programming a TV remote control and learning how to drive a car. Programming a remote control can certainly be a pain, but as long as you follow the instructions it can be completed. Now think about what happens when someone learns how to drive a car. While instructions on how to drive can teach you the basics, there are so many variables you can’t control, from icy roads to road construction to defensive driving. This results in no instructions covering it all.
So, the main difference between complicated and complex situations is that complicated situations can be well defined and have all possibilities accounted for while complex situations have so many changing variables that you can never account for them all. This doesn’t stop people from trying though with sometimes hilarious results.
For example, if you want to make an Elmo cake like the one below for a child’s birthday, it shouldn’t be that hard, right? There are plenty of places that can teach you the steps you need to do. For example, just follow the steps listed on this website.

However when you get done, it often comes out looking like this.

How does this happen? After all, the instructions were pretty clear about what the baker needed to do. The reality is that baking is a complex field that is often treated as if it’s complicated. Wouldn’t it be nice if we could all be Martha Stewarts and Rachey Rays just by following a set of instructions? Unfortunately, that’s not the way it works. There are so many variables that a set of instructions cannot easily address. For example, what about the temperature of the cake or the ratio of sugar to water in the frosting? Any of these variables could derail the process and mangle the final results.
Unfortunately, I think that this is also happening in math education when we help students to become better problem solvers.
I remember when I first started teaching mathematics in 2003, I was shown a poster much like the one below and loved it. The steps it listed seemed like the perfect solution to my students’ problem solving woes. At that time I thought that as long as students had these steps down, they were set.

Somewhere in the back of my mind, though, I knew something wasn’t right. While these steps did work for some problems, there were many problems where they completely fell apart. Fortunately I had the perfect solution for this issue: I stopped using math problems where this method did not work! It’s not my proudest moment, but we have to acknowledge where we’ve been to realize how far we’ve come.
The CUBES approach is well-intentioned, but it approaches problem solving like it was complicated and quickly falls apart if other variables are introduced. It overemphasizes computation and neglects helping students make sense of problems. It doesn’t really work for real life problems that are not in word problem format. If you’ve seen my video where I ask students about how old a shepherd is who has 125 sheep and 5 dogs, the final student mentions that she divided 125 by 5 because it didn’t say the sum, difference, or product. She is not a problem solver. She is just scanning for keywords she can box.
In contrast, let’s look at a complex approach to problem solving.
So, if the CUBES problem solving method isn’t ideal, then what should students use? Let me (re)introduce you to the Problem Solving Framework. This tool helps students think through the problem solving process using a method that works for almost all problems, even from different subjects like science and social studies! Take a look at the front and back of the Problem Solving Framework.
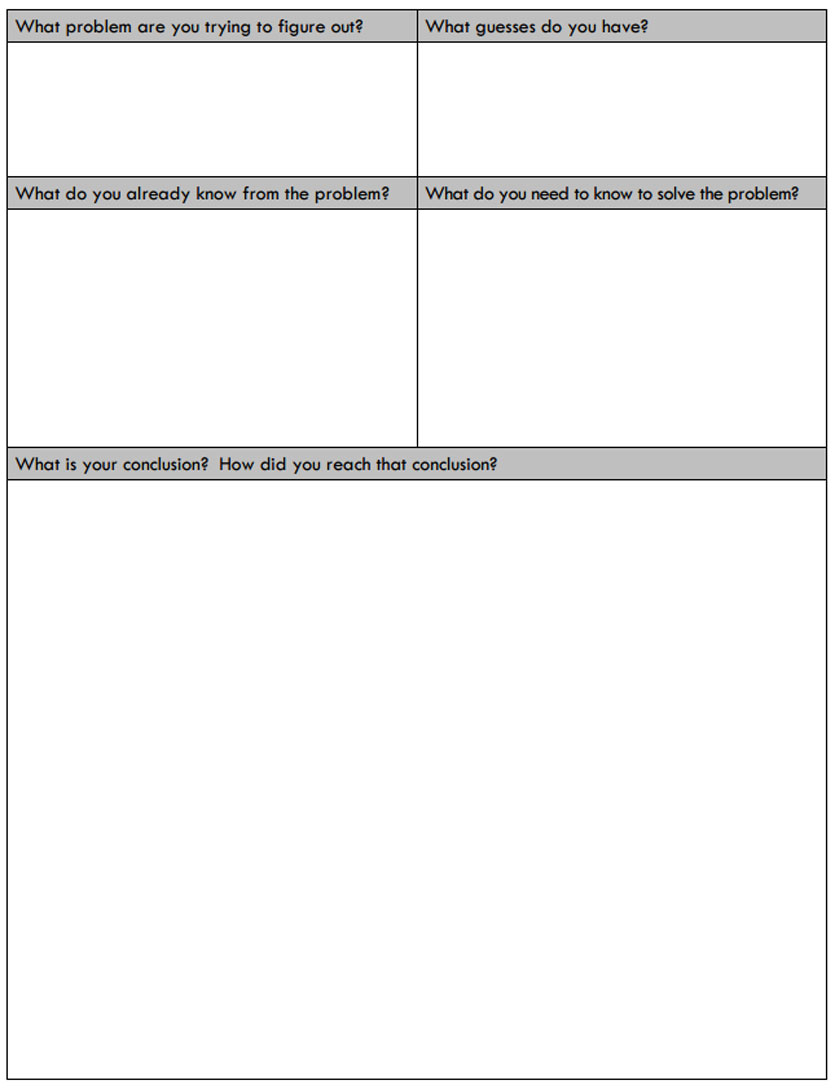

I talk about this tool in detail here, but the short version is that it takes students through a complex problem solving process including:
- Defining the problem
- Taking a guess
- Inventorying what information is already known or needs to be acquired
- Working on the problem
- Communicating their answer and how they reasoned about it
This process helps students solve most problems. It may not be as efficient as CUBES when it comes to simple word problems, but it doesn’t break down easily either. It emphasizes making sense of problems rather than jumping into computation. When students get lost during the middle of the problem solving process, you can have them walk through the steps they took, from reading the problem again to thinking about how they took the guess and what information they needed. This is often enough to get students back on track.
This is certainly not the only tool designed for complex problem solving. The Math Forum‘s “I Notice, I Wonder” format is another great option. The important part is to strike a balance between giving students enough structure to support their critical thinking, but not completely removing the need for it and skipping to the computation.
It is worthwhile to take a step back and reflect on how we are teaching our students to become better problem solvers. Complicated methods like CUBES may initially seem to work well, but aren’t long term solutions. Complex methods like using a problem-solving framework help students to deal with the variability of real problem solving. They don’t hold students’ hands as well, but they are truer to what problem solving is really about.
I hope this blog post has helped you reflect on your own practice. What am I right about? Where have I missed the mark? Please let me know in the comments.


The distinction between complicated and complex is very helpful and helps to clarify my own thinking. I’ve also not been a fan of these problem solving “methods” such as CUBES that you referenced here and you’ve really helped me to think through why.
Thanks Trevor. That’s one of the best compliments I can get for a post like this. That’s my entire hope. I think the complex and complicated framework makes it easier to have conversations about what makes strategies different from one another.
I work as a math coach for special ed teachers in my district and “CUBES” type methods are all pervasive in special ed. I’ll definitely be sharing your framework with these teachers especially as we push for a more complex curriculum for special ed students.
Still laughing. I think you’re going to get a lot of mileage out of this complex/complicated idea. I like the idea of the guess, because everyone can guess. It also provides a chance for students to discuss guesses at their tables and coming up with a “tabled best guess.”
Thanks Mike. Yeah, I do think comparing complicated to complex helps give language to differences we can feel but have trouble articulating. I hadn’t thought of it though beyond problem solving and depth of knowledge. I’ll keep my eyes open.
I highlighted the sentence, “The important part is to strike a balance between giving students enough structure to support their critical thinking, but not completely removing the need for it and skipping to the computation.” it really resonated with me. I’m going to share this post with the rest of my coaching team. Thanks!
Thanks Priscilla. Finding that balance is not easy… and I’ll probably spend the rest of my career fine tuning my teacher moves to get even better.
‘They don’t hold students’ hands as well, but they are truer to what problem solving is really about.’ This statement is relevant to what is happening in many classrooms, as teachers are trying to become ‘less helpful’. I enjoy your ideas and clarifications, and have shared your blogposts and lesson helps enough that my colleagues now follow and use you as a resource! I have already downloaded the ‘problem solver’ doc to use! Thank you!
Thanks Clara! I’m glad they have been useful and appreciate your support in sharing them with your colleagues.
I totally get that the “CUBES” and other strategies are over simplified. I have noticed that young math students (especially at underperforming/under-taught schools) need a structure to get started and remind them of all the parts of the problem. I have them draw or recreate the problem with materials and discuss the problem so they can visualize what needs to happen. AND they also need to work in small groups so we can discuss what they’re thinking and what makes sense. It needs to be fun cuz it is fun!! (and not to be only because they are tested… ad nauseum!). In essence we are working towards your format with guidance!
Thanks Penny. It sounds like you’re on the right track.
I like framing problem solving this way much better than some of the classic acronyms, and I like how you start your process with defining the problem and taking a guess. I wonder if “Inventorying what information is already known or needs to be acquired” could be broken down further into what information (e.g. the volume of an Olympic pool), skills (e.g. how to use the pythagorean theorem), and tools (e.g. ruler, graph paper, desmos) might be needed.
I also wonder if there might need to be a step before “working on the problem” that involves planning through the problem solving process. I feel that since problem-solving can be so complex, students should be reminded to take some time to think about their approach before starting work on the problem. Although the approach might be different in different situations (which is why CUBES often fails), it’s probably worth it to at least have a plan (even if it gets changed later). Thoughts?
Thanks Bob. What you’ve shared makes sense. In my experience, this has worked out well for me, but it isn’t to say that it can’t be improved (after all, it’s on version 8.1 anyway!). I guess it comes down to a balance between too much and too little direction. From my experience, students infer much of what you suggest. Give it a shot though and see what you learn, then let me know.
I think you hit the nail on the head with the difference between complicated and complex. It also confirms why prefilling the “What problem are you trying to figure out?” box would be a HUGE mistake. Again, it’s all about creating the habit so that students will know what to do even when they don’t have PSF handed to them at some point.
BTW, I love the analogy of cooking being complex! Passing along recipes to my young adult boys when they went off to college was interesting. They learned to cook with me while in high school, but I would get phone calls asking what various instructions mean as they tried following recipes on their own.
Thanks Michele. I really love how comparing complex and complicated makes this so much easier to unpack.
Complex and complicated are definitely words that I will in future be sure to assign accurately when describing a problem.
From prior experiences when interacting with teachers during maths PD. I have noticed that teachers start doing that mumbly, non-committal, type answer when problems are more complicated than complex. Personally I also feel more confident in my abilities to attempt a complex problem than a complicated problem. Give’s me more insight into how my students may also be feeling. I think I should explain to them why the problem is either more complex than complicated, it may help them to find a starting point of where to begin to solve the problem- and define these terms of course.
I came across this recently, https://www.mathcoachminute.com/post/define-explore-solve. It’s another alternative to CUBES. What is your opinion of this?
Thanks for asking. The short version is that while I’ve never seen this before, nothing seems bad about it.
The longer version is that I ask myself, could this actually work in real life? The DEFINE part is absolutely what we do in real life: we try to figure out what the heck we’re even doing. So I love that and I have something similar in the Problem Solving Framework.
The EXPLORE part is helping them think about ways to problem solve. It’s not holding their hand, for better and for worse. Could it be more specific? Maybe. Could being more specific be a bad thing? Maybe.
The SOLVE part is again kinda vague but I’d definitely prefer to it be vague than be like CUBES where it is WAY too specific. So yeah, I don’t have a problem with it but I also don’t know how useful it would be either. So it’s not quite an endorsement but certainly it’s not something I’d condemn.
Thank you for your thoughtful reply. I see what you are referring to in the SOLVE portion. I plan to spend some time with this over the summer.