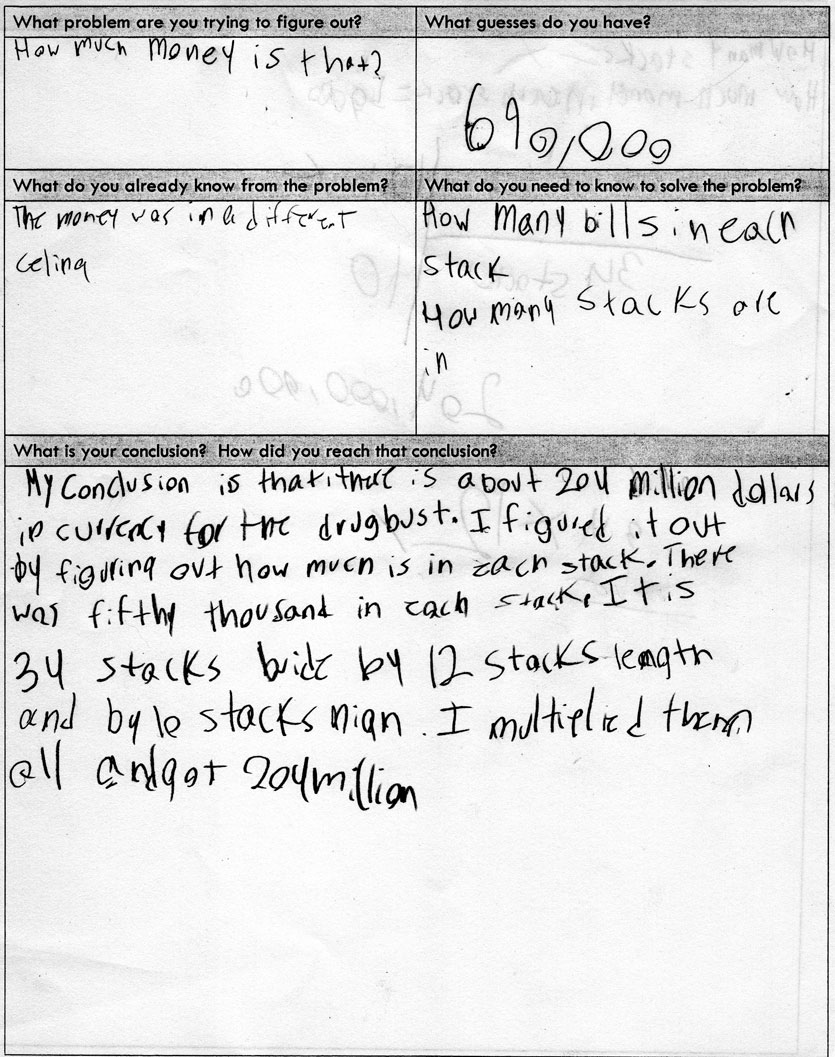
- How much money is that?
- What is a guess that is too low?
- What is a guess that is too high?
- What is your best guess?
- What options do you have for counting this money and what are the advantages and disadvantages to each method?
- How can we measure the volume of $100 bills in the pile?
- How important is it that we have an accurate answer?
Picture 1

Picture 2

Picture 3

A few notes about the money:
- My assumption is that all of the United States bills are $100 bills.
- I am ignoring the colorful money in front of the United States bills as they do not appear to be US currency.
- Looking at Picture 1 (with help from Picture 3) it looks like the main stack of bills go back 11 rows with a slightly less high 12th row in the front.
- Looking at Picture 1 (with help from Picture 2) it looks like the pile has 34 columns of bills.
The most important part of this lesson will be the mathematical modeling conversation regarding how to go about counting the money. So, after getting students’ guesses, be sure to have a long talk around “What options do you have for counting this money and what are the advantages and disadvantages to each method?” Here are my thoughts on what students might say:
- You could count each bill, one at a time.
- Advantage: very accurate if you don’t lose track of the running total
- Disadvantage: very time consuming
- You could count the bills using an electric bill counter (as clearly you would be able to afford one!)
- Advantage: potentially the most accurate if you don’t lose track of the running total
- Disadvantage: also very time consuming as you can only put stacks of up to 130 bills at a time
- You could hire multiple people to count them for you.
- Advantage: potentially faster than the previous two methods but still time consuming
- Disadvantage: they could steal your money and you have to pay them
- You could estimate how much money is in the pile based on its weight
- Advantage: should take less time than counting the individual bills
- Disadvantage: limited accuracy as the scale may not be precise and the bills’ weight may change from handling
- You could estimate how much money is in the pile based on its volume
- Advantage: by far the fastest method
- Disadvantage: limited accuracy
Ultimately you should guide students to see that while the first four options are reasonable, the only one we can complete based on the information we have is option 5 where we estimate how much money there is based on its volume. This is an important opportunity for students to build conceptual understanding of volume instead of skipping straight to procedures. Give students the images and let them strategize about “How can we measure the volume of $100 bills in the pile?” There are quite a few options including the ones below so help guide students to see the connections between them:
- Find the amount of money in one stack of bills and multiply it by the number of stacks.
- Find the amount of money in one layer of bills and multiply it by the number of layers.
- Find the amount of money in one row of bills and multiply it by the number of rows.
- Find the amount of money in one column of bills and multiply it by the number of columns.
- Find area in square units of one layer of bills and divide it by the area of one bill, then multiply it by the number of layers.
It is worth noting the the first four options are the easiest and do not use the traditional volume procedure of measuring the length, width, and height in linear units and multiplying them all together. Regarding the last option above, it is one of the few I could think of that begins with such an approach.
In terms of coming up with the estimate, here is how I approached it:
- Main pile of money:
- There are 10 rows and 34 columns of money in the main pile so there are 340 stacks of money.
- To estimate height, the largest stack of paper whose count I know are reams of paper. I estimate that highest stacks of money are as tall as 15 reams of paper.
- Reams of copy paper hold 500 sheets while reams of cardstock often hold 250 sheets. The money itself is used and likely crinkled so I estimate that it is between the thickness of copy paper and cardstock but closer to copy paper. I estimate that a stack of used bills that are the same height as a ream of paper is 400 bills.
- Multiplying this all together: 340 stacks * 15 reams tall * 400 bills per ream * $100 per bill gives a total for the main stack of $204 million
- Shorter single row of bills in the front
- I can see from Picture 2 that it is also 34 columns across giving us 34 stacks of bills.
- The stacks on the left half appear to be about two reams of paper shorter than the main pile of money behind it.
- The stacks on the right half appear to be about two reams of paper short than the left half.
- Averaging the two I get that they are about 12 reams of paper tall.
- Multiplying this all together: 34 stacks * 12 reams tall average * 400 bills per ream * $100 per bill gives a total for the main shorter single row of bills of $16,320,000.
- Thus, the grand total I have is $220,320,000.
Once students have their estimates in, play this clip for them. Note that there is such a large margin of error in their estimates that the news anchor says “200 million” while the text says “205 million.
Make sure to have students discuss why they think their answer was different from what the news anchor stated. For example, I think my answer was a little high because of my estimation of the number of bills in each stack. If I had estimated that there were 372 bills per ream, I would have been right on the money.
According to this article from the Los Angeles Times, they state more specifically that there was $205.6 million in cash. Other interesting details they include are:
- “the cash seized was mostly in U.S. $100 bills and weighed at least 4,500 pounds.”
- “experts were still analyzing the $205.6 million in cash to check for counterfeits but that the bills appeared to be legitimate.”
- “Officials with the attorney general’s organized crime unit used a moving truck, guarded by a 25 patrol-car caravan, to take the money to its headquarters.”
- Low (wrong answer, wrong strategy)

- Medium (wrong answer, mostly right strategy – 2,805,000 is the quantity of $100 bills, not the value of the $100 bills)

- High (relatively right answer, right strategy)
- CCSS 5.MD.3 Recognize volume as an attribute of solid figures and understand concepts of volume measurement.
- CCSS 5.MD.4 Measure volumes by counting unit cubes, using cubic cm, cubic in, cubic ft, and improvised units.
- CCSS 5.MD.5 Relate volume to the operations of multiplication and addition and solve real world and mathematical problems involving volume.
- CCSS 5.MD.5b Apply the formulas V = l × w × h and V = b × h for rectangular prisms to find volumes of right rectangular prisms with whole-number edge lengths in the context of solving real world and mathematical problems.
- CCSS 5.MD.5c Recognize volume as additive. Find volumes of solid figures composed of two non-overlapping right rectangular prisms by adding the volumes of the non-overlapping parts, applying this technique to solve real world problems.
- CCSS 6.G.2 Find the volume of a right rectangular prism with fractional edge lengths by packing it with unit cubes of the appropriate unit fraction edge lengths, and show that the volume is the same as would be found by multiplying the edge lengths of the prism. Apply the formulas V = l w h and V = b h to find volumes of right rectangular prisms with fractional edge lengths in the context of solving real-world and mathematical problems.
- CCSS 7.G.6 Solve real-world and mathematical problems involving area, volume and surface area of two- and three-dimensional objects composed of triangles, quadrilaterals, polygons, cubes, and right prisms.
- CCSS G-MG.1 Use geometric shapes, their measures, and their properties to describe objects (e.g., modeling a tree trunk or a human torso as a cylinder).



5 Comments