Today we found this problem on the Smarter Balanced Practice Test (Grade 8 – Question 15):
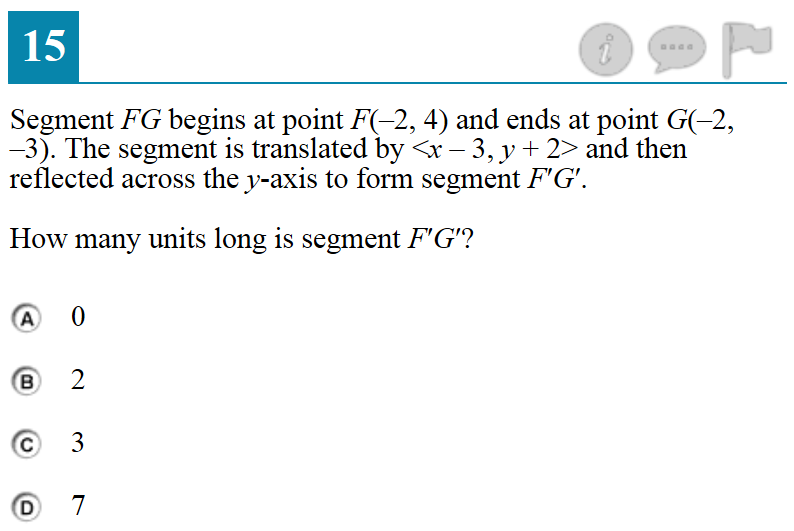
It provided the basis for a worthwhile conversation on the importance of students having conceptual understanding. The problem is based on these 8th grade standards:
There are two main ways to approach this problem: conceptually or procedurally.
If you have conceptual understanding of transformations, then you understand that rotating, reflecting, or translating a line segment doesn’t change its length. Dilating a line segment may change its length, however this problem only mentions a translation and reflection so the length will be preserved. The line segment is initially 7 units long, so regardless of how it is translated and reflected, it will remain 7 units long. This problem then takes less than a minute to complete.
If you have procedural skill and fluency but no conceptual understanding of transformations, then you are forced to perform all of the time-consuming instructions. After translating the points from F(-2, 4) and G(-2, -3) by <x – 3, y + 2>, the new points will be F'(-5, 6) and G'(-5, -1). Then you have to reflect the new points across the y-axis taking F’ and G’ to F”(5, 6) and G”(5, -1). Now we can measure the distance between the two points and see that they are still seven units apart. While this approach may not be much more difficult, it is not as efficient as the conceptual approach. Also, consider how much more difficult it would have been if a 30 degree rotation was added to the instructions.
We appreciated this opportunity to reflect on conceptual understanding’s significance.


My school is currently having a debate about the place of motion rules in the 8th grade transformation standards. We pay for the Ready curriculum, and we’ve looked through a number of PARCC (we are a PARCC state) sample items, this years MCAS (MA state assessment) and more resources. We’ve found nothing about motion rules (rules of the form (x+3, y-2) for a translation, or (-x, y) for a reflection across the y-axis). I looked through the rest of the SBAC module, and didn’t see any other examples of this. What do you think?
Thanks for your comment Dylan. I believe that topic falls under G-CO.2 which states “describe transformations as functions that take points in the plane as inputs and give other points as outputs.” Perhaps there are no good examples to be found, but I feel confident it will be assessed eventually.
Thank you for sharing this! I am in a PARCC state, and there is only so much time for being able to look at resources for both PARCC and Smarter Balanced, so I’m glad to see this question through your blog. What a great example to be able to share with teachers and students.
Thanks Jennifer!