The Art of Questioning in Mathematics

My best advice to anyone preparing to implement the Common Core State Standards (CCSS) for mathematics is to get students talking and writing about their work.  Regardless of your stance on the CCSS, being an effective communicator is a valuable and often challenging life skill.  It is even more challenging when the content is not something you feel completely comfortable with.  You can be certain that the CCSS assessments will have plenty of questions where students will be required to articulate themselves mathematically.  Consider the similarities and differences between each problem in the two sets below.
 

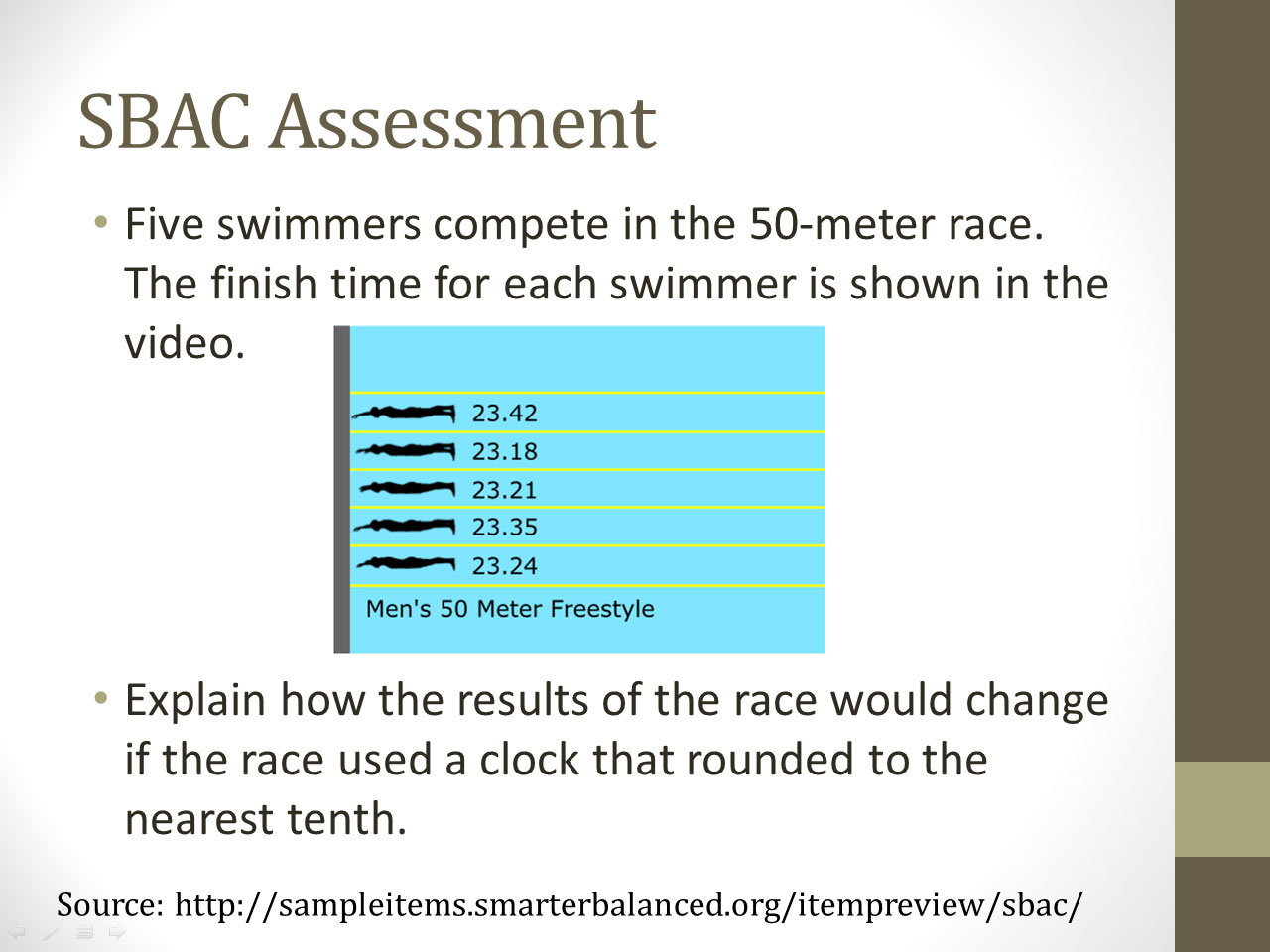
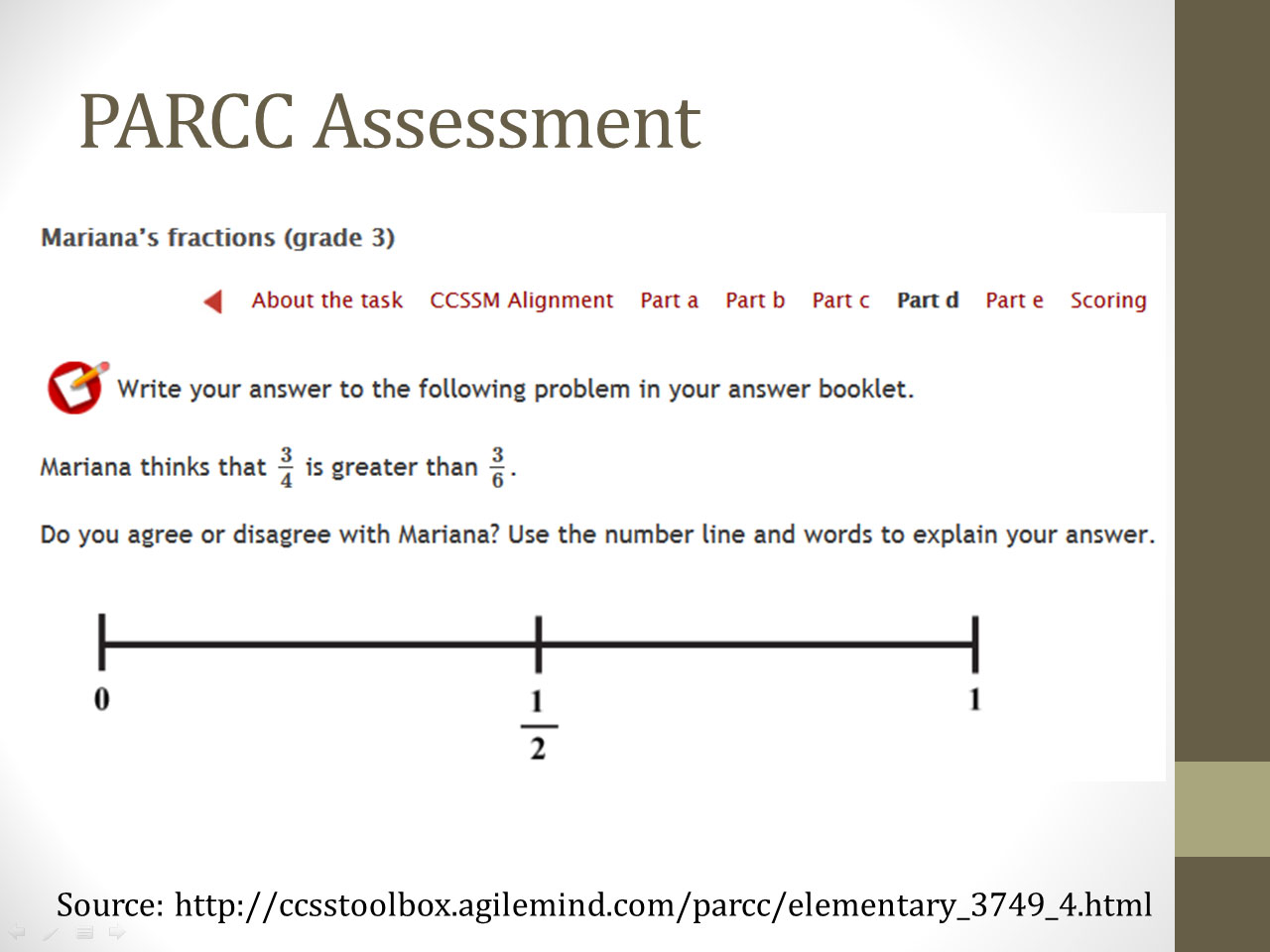
In both questions from the outgoing state assessments, students were asked multiple choice questions where no explanation was required.  Now students will be expected to find the answer and explain themselves using sentences.

Fortunately, there are many things mathematics teachers can do to get students comfortable with explaining their thinking.  If you’ve seen my lessons, then you know that one of my favorite methods is providing students with a problem in a context that interests them.  The right context will engage them so thoroughly that it will be a challenge to get them to stop telling you what they think.

Another extremely valuable approach is improving the questions that teachers ask.  Teachers are aware that some questions require students to think more meaningfully, yet research shows that these questions are rarely asked.  Meredith D. Gall stated in The Use of Questions in Teaching that “about 60% of teachers’ questions require students to recall facts; about 20% require students to think; and the remaining 20% are procedural.”

So if our goal is to increase the percentage of our questions that “require students to think,” where do we begin?  We know that students often have an easier time explaining themselves orally before they can articulate themselves in writing.  Can you think of questions that you do/could/should ask that would get them to do this?  How realistic is it to come up with a question on the fly with limited classroom minutes?

I have faced the same struggles and I want to share a resource that is very useful in increasing the percentage of questions that “require students to think.”  Below is a version of The Art of Questioning in Mathematics that have been modified from the NCTM Professional Teaching Standards.   It contains questions that teachers can ask to further students’ thinking.  Some suggestions for incorporating these questions include:

• Write them into a lesson plan
• Put them on a podium/clipboard
• Print them out in large letters and place around the room to remind you

You can download a PDF copy by clicking on the “Download PDF” button.  I have underlined some of my favorite questions.
 

• “What do others think about what____________said?”
• “Do you agree? Disagree? Why or why not?”
• “Does anyone have the same answer but a different way to explain it?”
• “Would you ask the rest of the class that question?”
• “Do you understand what they are saying?”
• “Can you convince the rest of us that that makes sense?”

• “Why do you think that?”
• “Why is that true?”
• “How did you reach that conclusion?”
• “Does that make sense?”
• “Can you make a model and show that?”

• “Does that always work? Why or why not?”
• “Is that true for all cases? Explain?”
• “Can you think of a counter example?”
• “How could you prove that?”
• “What assumptions are you making?”

•  “What would happen if ____________? What if not?”
• “Do you see a pattern? Explain?”
• “What are some possibilities here?”
• “Can you predict the next one? What about the last one?”
• “How did you think about the problem?”
• “What decision do you think he/she should make?”
• “What is alike and what is different about your method of solution and his/hers?”

• “How does this relate to __________?”
• “What ideas that we have learned before were useful in solving this problem?”
• “Have we ever solved a problem like this one before?”
• “What uses of mathematics did you find in the newspaper last night?”
• “Can you give me an example of ___________?”