How Many Gumballs Fit In The Gumball Machine?

 
“At 79 inches tall (6’10”), our Jumbo Giant Gumball Machine is one HUGE gumball machine that will tower over children and adults alike! This gumball machine has a massive globe that can hold ##,### gumballs, but comes with a smaller inner globe to allow you to stock it with ##,### gumballs to save money and still make it look full (inner globe takes up space and pushes gumballs to outer globe). This is the same giant gumball machine seen in arcades, amusement parks, and shopping malls.” (Gumballs.com)
 

• How many gumballs fit in the gumball machine?

 

These questions may be useful in helping students down the problem solving path:

• What information would be useful in figuring this out?
• What factors may affect your answer’s accuracy?
• What is a guess that is too low?
• What is a guess that is too high?

 

This problem will range in difficulty depending on what questions are asked and what assumptions are made (such as whether to assume an inner globe is included).  In general, I have found this problem to have four stages.

1. Students find the volume of the large outer globe (assuming no inner globe) as 22,000 cubic inches.
2. Students find the volume of the gumball (assuming 1″ diameter) as ~0.5 cubic inches.
3. Students divide the volume of the larger outer globe by the volume of the gumball to get ~45,000 gumballs.
4. Students realize that ~45,000 gumballs is too many and try various strategies to lower the amount.

 

Some common student errors include students who think the gumball’s diameter is 1 cubic inch because 1 x 1 x 1 = 1.  That would be true for a 1″ cube shaped gumball.  Also, students have issues with units and this is a good opportunity to discuss the difference between inches, square inches, and cubic inches.
 

• Gumball machine info with data covered:

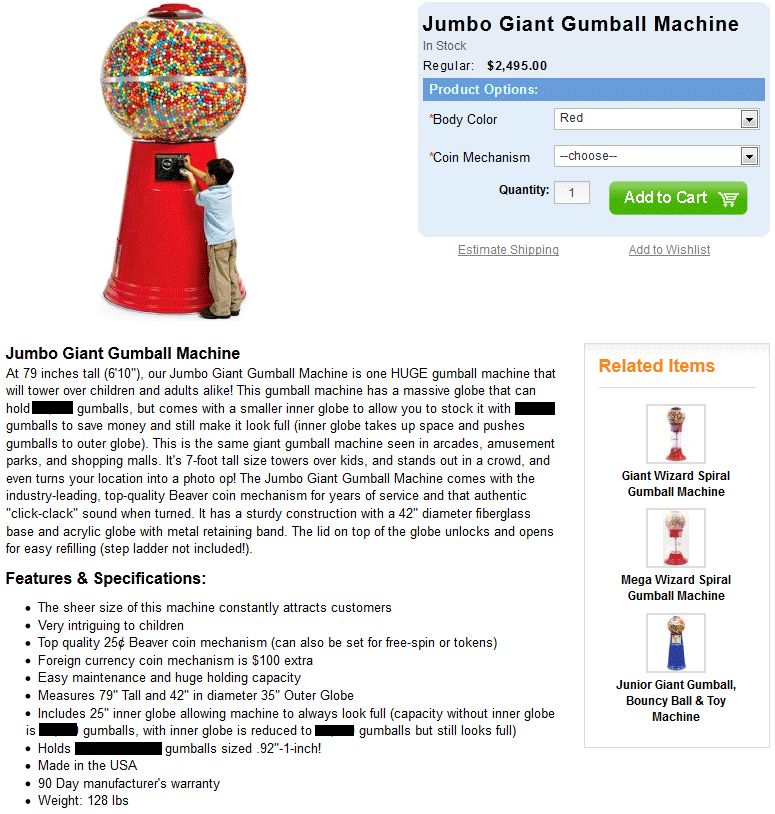
• Gumball machine info with data showing:

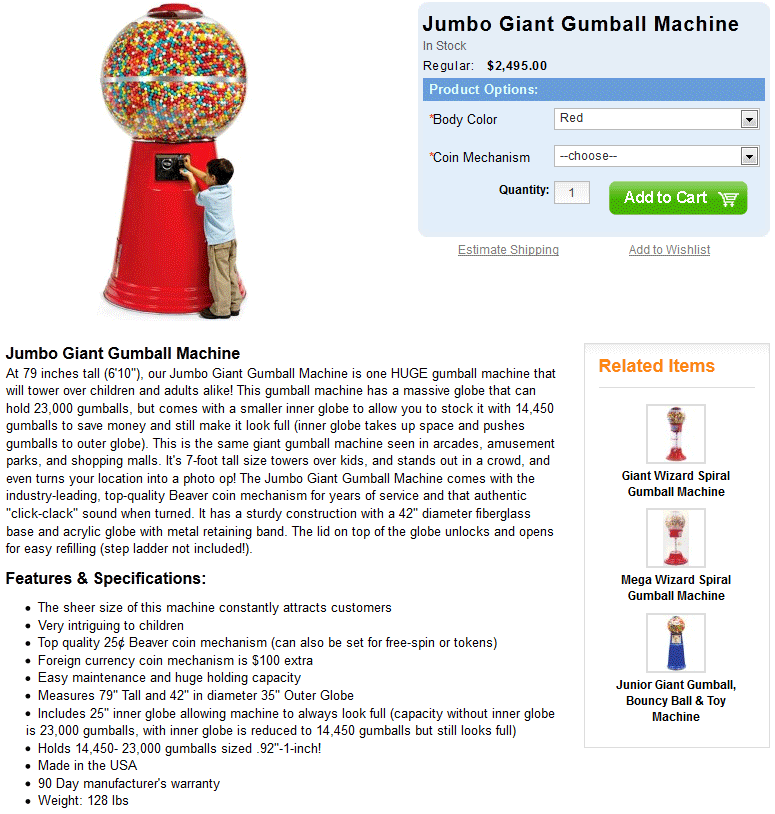
• Gumball machine:

Below are medium and high work samples.  The medium work sample has a reasonable answer but the explanation is more calculation based than context based and is not coherent enough.

• Medium

• High

• High

• High

 

• CCSS 8.G.9 Know the formulas for the volumes of cones, cylinders, and spheres and use them to solve real-world and mathematical problems.
• CCSS G-GMD.3 – Use volume formulas for cylinders, pyramids, cones, and spheres to solve problems.

 

• 101questions
• Gumballs.com
• Amazon.com