How Big Is The 2010 Guatemalan Sinkhole?

 
In 2010, an enormous sinkhole (pictured below) suddenly appeared in the middle of a Guatemalan neighborhood and swallowed a three-story building above it.
 

• How much material will they need to fill the sinkhole?
• How much will it cost to fill the sinkhole in?

 

These questions may be useful in helping students down the problem solving path:

• What is a guess that is too low?
• What is a guess that is too high?
• What is your best guess?
• What information do we need to know to figure this out?

 

The first thing a person should think when they see this photo is “There is no way this is real!” or “This is totally PhotoShopped!”  That is what makes the photo so amazing because it is completely real and documented by many reputable sources such as National Geographic, Time Magazine, and CNN.  Each of these articles can explain why and how the sinkhole (technically called a “piping pseudokarst“) happened better than I can, so I will focus on the math involved.

One of the sinkhole’s amazing properties is how incredibly cylindrical it is.  The other thing I find striking is how deep it appears.  It looks like it goes down and never stops.  I wouldn’t be surprised if students thought it might go all the way through the Earth and come out on the other side (which apparently is not China but actually in the middle of the Indian Ocean).  In actuality it does stop and this video below shows that better.

All the challenges revolve around knowing the sinkhole’s dimensions, however its reported dimensions vary.  You can have students discuss the three reported options and debate to find the one that is most reasonable or skip this step and go with CNN article I have included (which I believe has the most accurate dimensions):

• National Geographic: “60 feet (18 meters) wide and about 30 stories deep” (assuming a story is about 10 feet, then about 300 feet deep)
• Time Magazine: “runs some 200 ft. deep”
• CNN: “The 20-meter (about 66 feet) diameter sinkhole is about 30 meters (about 100 feet) deep.”
• Slate: “A sinkhole, 65 feet across and 100 feet deep”

I think it is worth having students reflect on how reasonable these estimates are.  Here are some of my thoughts:

• National Geographic, CNN, and Slate seem to agree that the diameter is about 20 meters, so that is what I will assume.
• The depth measurement varies from 100 feet to 200 feet to 300 feet.  Obviously that is a huge difference.
• The pictures don’t seem to be helpful in determining the depth.
• Looking at the video, the sinkhole looks to be a little more than 1.5 times deeper than it is wide.  So, that seems to match up with the CNN and Slate measurements.

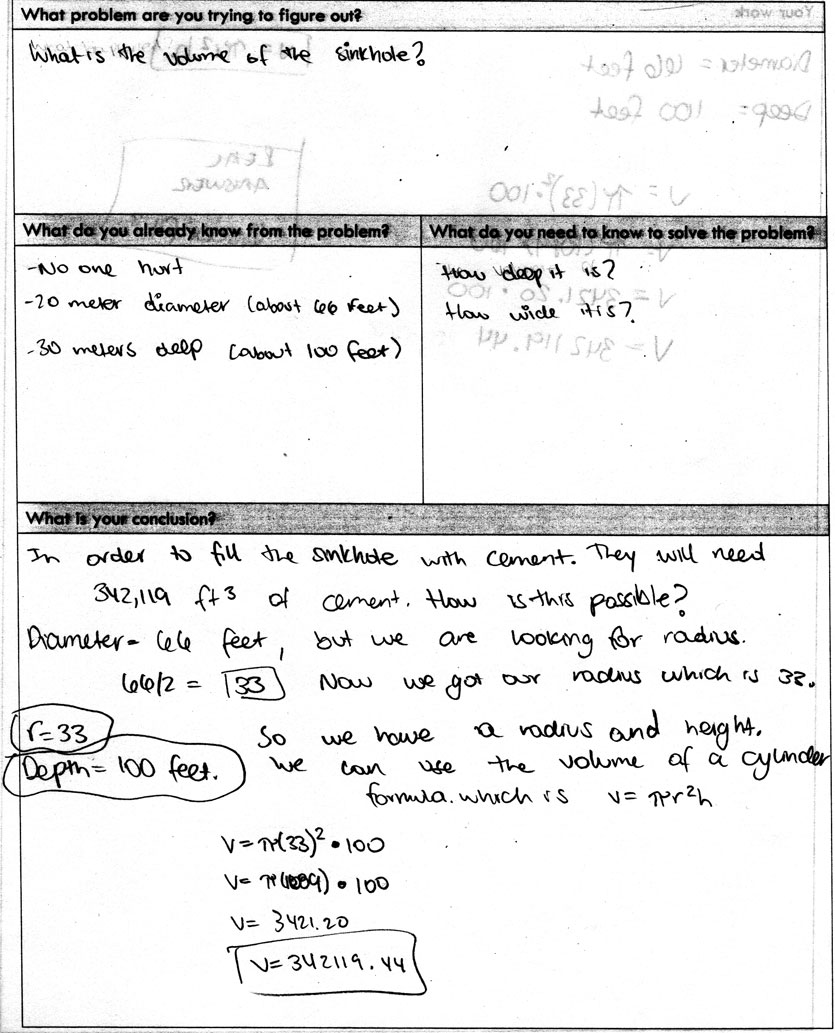
Going forward with dimensions of diameter of 66 feet and depth of 100 feet, we can use the formula for the volume of a cylinder: pi * radius^2 * height.  That gives us pi * 33^2 & 100 cubic feet or approximately 342,119.45 cubic feet.

Interestingly, Slate appears to have made a mathematical error in their reporting (below)

The only way I see how they could get 6,500 cubic feet as an answer using their reported dimensions of 65 feet in diameter and 100 feet in depth is to multiply 65 by 100.  Clearly that is not correct.  This is a great opportunity for students to double check the validity of their answer and defend their reasoning.

Update: I actually contacted the article’s author and he replied “Apparently you picked the wrong article for a math lesson! I apologize. It appears you are correct.”  So, it is an example of how even professionals make silly mistakes and how we have to check our work to make sure it is reasonable.  Using low, high, and best guesses is helpful for this.

The next challenge is “How much will it cost to fill the sinkhole in?”.  Obviously a lot of assumptions need to be made.  Slate reported that in 2007 Guatemala filled a larger sinkhole (about 330 feet deep) with concrete.  So, using that information:

A cubic foot of concrete currently costs about $4.  $4 * 342,119.45 costs about $1,368,477.80.  That obviously does not include labor, material transportation, or really anything besides the concrete itself.  The price of concrete also varies, so it would be reasonable for the total cost to be somewhere between $1 and $2 million.  In the Slate example, the previous sinkhole cost $2.7 million so this seems like a reasonable estimate.
 

• Wide view of the sinkhole and the city

• View of the sinkhole with the helicopter’s shadow in the hole.

• Close view of the sinkhole

• Close view of the sinkhole

• Close view of the sinkhole

Below are medium and high work samples for the challenge.  The only issues I had with the medium work samples were missing or incorrect units.

• Medium

• Medium

• High

• High

• High

 

• CCSS 8.G.9 – Know the formulas for the volumes of cones, cylinders, and spheres and use them to solve real-world and mathematical problems.
• CCSS G-GMD.3 – Use volume formulas for cylinders, pyramids, cones, and spheres to solve problems.
• CCSS G-MG.1 – Use geometric shapes, their measures, and their properties to describe objects (e.g., modeling a tree trunk or a human torso as a cylinder).

 

This problem would not exist without Katie’s submission on 101qs.com (a website created by Dan Meyer).  This picture was begging to be explored in more depth (no pun intended) and led to this lesson.  Thanks to both of them and all math educators who share their hard work.
 

• 101qs.com
• National Geographic
• Time Magazine
• CNN
• Slate
• Wikipedia
• What is on the other side of the world from Guatemala?
• YouTube
• Location of sinkhole on Google Maps