When the Common Core State Standards (CCSS) for Mathematics were written, the authors defined three shifts (coherence, focus, and rigor) to articulate “how the standards differ from previous standards—and the necessary shifts they call for.” The shifts are useful in articulating the big picture as to how things have changed. This blog post delves solely into the coherence shift.
The official CCSS website lists this explanation for the coherence shift:
Coherence: Linking topics and thinking across grades
Mathematics is not a list of disconnected topics, tricks, or mnemonics; it is a coherent body of knowledge made up of interconnected concepts. Therefore, the standards are designed around coherent progressions from grade to grade. Learning is carefully connected across grades so that students can build new understanding onto foundations built in previous years. For example, in 4th grade, students must “apply and extend previous understandings of multiplication to multiply a fraction by a whole number” (Standard 4.NF.4). This extends to 5th grade, when students are expected to build on that skill to “apply and extend previous understandings of multiplication to multiply a fraction or whole number by a fraction” (Standard 5.NF.4). Each standard is not a new event, but an extension of previous learning.
Coherence is also built into the standards in how they reinforce a major topic in a grade by utilizing supporting, complementary topics. For example, instead of presenting the topic of data displays as an end in itself, the topic is used to support grade-level word problems in which students apply mathematical skills to solve problems.
The most important takeaways I read from this description are that coherence is built in both vertically (topics are coherently connected across the grades) and horizontally (topics are coherently connected with a single grade) . While this is a useful explanation, I find it helpful when working with teachers to share additional specific examples of what this looks like.
To describe what vertical coherence looks like, I use the slide below that depicts how students’ ability to solve real-world geometry problems grows from elementary school through high school.
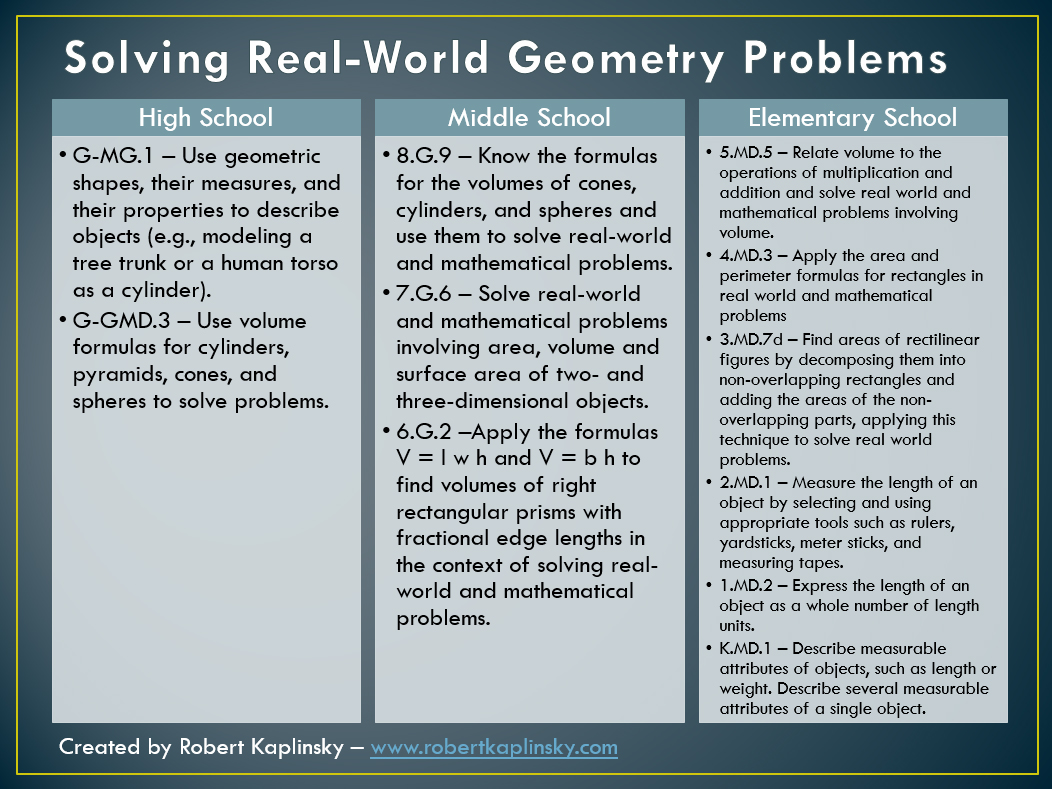
In early elementary school, students begin by measuring objects’ attributes and move into measuring length. This provides the foundation that most real-world geometry problems will be built upon.
- K.MD.1 – Describe measurable attributes of objects, such as length or weight. Describe several measurable attributes of a single object.
- 1.MD.2 – Express the length of an object as a whole number of length units.
- 2.MD.1 – Measure the length of an object by selecting and using appropriate tools such as rulers, yardsticks, meter sticks, and measuring tapes.
By upper elementary school, students are building upon their knowledge of length and are now measuring area and beginning with volume. Note how each standard includes applying or solving real world or mathematical problems.
- 3.MD.7d – Find areas of rectilinear figures by decomposing them into non-overlapping rectangles and adding the areas of the non-overlapping parts, applying this technique to solve real world problems.
- 4.MD.3 – Apply the area and perimeter formulas for rectangles in real world and mathematical problems
- 5.MD.5 – Relate volume to the operations of multiplication and addition and solve real world and mathematical problems involving volume.
In middle school, students are continuing their work with volume and including surface area to solve more complex real world problems. Note how the standards continue to discuss applying or solving real world or mathematical problems.
- 6.G.2 –Apply the formulas V = l w h and V = b h to find volumes of right rectangular prisms with fractional edge lengths in the context of solving real-world and mathematical problems.
- 7.G.6 – Solve real-world and mathematical problems involving area, volume and surface area of two- and three-dimensional objects.
- 8.G.9 – Know the formulas for the volumes of cones, cylinders, and spheres and use them to solve real-world and mathematical problems.
By the time the students reach high school, their ability to solve real world or mathematical problems has been built upon for many years. Now students are expected to apply their cumulative knowledge to all situations. Also note the difference between 8.G.9 and G-GMD.3. Both involve solving problems that require the volume formulas for cones, cylinders, and spheres. The main difference comes from that first verb where in 8th grade a lower level of taxonomy is expected with “know” the formulas while in high school students are expected to “use” the formulas.
- G-MG.1 – Use geometric shapes, their measures, and their properties to describe objects (e.g., modeling a tree trunk or a human torso as a cylinder).
- G-GMD.3 – Use volume formulas for cylinders, pyramids, cones, and spheres to solve problems.
Together this chain of standards illustrates the coherent progression students experience to increase their ability to apply mathematics from elementary school through high school. With an example of this vertical coherence defined, let’s look at what horizontal coherence looks like.
Using 8th grade as an example, there is a clear emphasis on the standards’ interconnected nature. Consider these three standards that come from the domains expressions & equations, functions, and statistics & probability:
- 8.EE.5 – Graph proportional relationships, interpreting the unit rate as the slope of the graph. Compare two different proportional relationships represented in different ways. For example, compare a distance-time graph to a distance-time equation to determine which of two moving objects has greater speed.
- 8.F.3 – Interpret the equation y = mx + b as defining a linear function, whose graph is a straight line; give examples of functions that are not linear. For example, the function A = s2 giving the area of a square as a function of its side length is not linear because its graph contains the points (1,1), (2,4) and (3,9), which are not on a straight line.
- 8.SP.2 – Know that straight lines are widely used to model relationships between two quantitative variables. For scatter plots that suggest a linear association, informally fit a straight line, and informally assess the model fit by judging the closeness of the data points to the line.
These standards emphasize that students should be connecting the ideas of:
- proportionality
- unit rates
- slope
- linear equations
- scatter plots
- lines of best fit
I have taught all of these standards before but I hadn’t emphasized how they coherently relate to each other. Slopes of linear equations represent a constant unit rate that is proportional across the line. Scatter plots with a correlation are more easily represented with a line of best fit which even ties in statistics. Taught together in eighth grade, these topics form a coherent message about mathematics’ interconnected nature. What other examples of CCSS coherence do you see?
Click the button below to download a PDF version of the “Solving Real-World Geometry Problems” slide.

I wonder if creating a word cloud out of all of the math standards (or any subject for that matter) to post in the room would serve as a visual reminder to teacher and students of just what you point out: the key ideas that should be connected throughout the year-long study. Not the most rich or deep thing to do, but a place to start? Off to test this and see what it would look like for fourth grade!
Tried it. Not great. Can you think of ways that you would recommend teachers identify and highlight connection points? Is this something you sit down and tackle at the beginning of the year? Semester? Keep as a list on your board?
My recommendation would to really walk teachers through the entire progression. In some ways teachers “know” how to find the area, surface area, or volume, but I think that “know” is a relative term.
I “knew” how to find the area because I could plug numbers into the formula, but I had absolutely no clue where many of the formulas came from. For example, I was absolutely shocked that there was actually a reason for the area of a circle being pi x radius^2. It didn’t even occur to me that there could be a reason for that.
So, that begs the question of how deeply someone has to understand the material to really see the connections. I would say that if you can us the procedure, but you don’t know where the formula comes from, that’s a pretty good sign that you don’t see the connections. Again, I know this from first hand experience.
I didn’t even realize the connection between nets of 3D shapes and surface area! I was that much of a robot. So, that’s where I would begin.
Just because there is coherence among grades doesn’t always mean the standards work that well. This one of those cases I dislike. The focus on volume and surface area starts occurring so early that its very hard to motivate why the formulas work. This progression tends to encourage the kind of thinking you mention at the start of the mail “there is a lot of math that I can do without actually understanding why it works.
What would you do differently, Benjamin?
I think I would delay surface area and most volumes until there is enough conceptual framework to motivate them and concentrate on other early geometric ideas instead. For example: developing similarity and geometric transforms in the context of upper Elementary and MS makes more sense to me or looking at tiling or even playing more generally with folding/origami.
That could very well work. I wonder when the next rethinking of state standards will take place and how collaborative that process will be.
8.G.9 – Know the formulas for the volumes of cones, cylinders, and spheres and “use” them to solve real-world and mathematical problems.
G-GMD.3 – Use volume formulas for cylinders, pyramids, cones, and spheres to solve problems.
From your writing…”Also note the difference between 8.G.9 and G-GMD.3. Both involve solving problems that require the volume formulas for cones, cylinders, and spheres. The main difference comes from that first verb where in 8th grade a lower level of taxonomy is expected with “know” the formulas while in high school students are expected to “use” the formulas.”
Doesn’t the second part of 8.G.9 ask students to “use” the formulas rather than just “know” them? Am I over-thinking this 8th grade standard?
To be honest Tabatha, now that you mention this, you make a great point. I do wish there was more clarity here. Sometimes I feel like I’m interpreting the wrinkles on a hand when reading the standards.
I think that in the early grades, the formula is not the thing. My understanding is that the fifth grade standard is about packing layers of cubes into rectangular prisms so one can figure out that one must multiply the three dimensions as a shortcut to finding the volume, because you multiply the adjacent sides of the base rectangle to get its area, then multiply by the height which is how many layers of that area. This leads to V=Bh. In sixth grade, students continue to explore volume of rectangular prisms by trying to figure out how many cubes of FRACTIONAL side lengths can be packed into the prism. If the cubes are 1/2″ on each side how many cubes will fit into a given prism. As students learn volume that is necessary in later grades, they start with a conceptual understanding. Formulas are an extension of that understanding.
I feel that elementary grades spend more time on the conceptual and reprentational side of the geometry standards so that students can visually undetstand the concept mak8ng it easier in the upper grades to understand the abstract concepts.
Are you able to remember all of the meals you had during this week, two years ago? Unless you eat the same thing, repeatedly, it might be a little difficult. The 8th grade geometry standards are taught and not revisited until Geometry, which is often taught two years later. What do we do for topics taught in such isolation? Revisit them in Algebra I, when we already have a tremendous amount to cover?
I don’t have a great answer for this question, Jim. I too forget so much that I learn and never use again. It does make me wonder about this thought experiment:
If K-12 math standards did not exist anywhere, and tomorrow they would be created, how would they be different than what we have now? What would go away? What new standards would we have?
To answer your closing question, I feel that one of my favorite examples of coherence that went unmentioned in your 8th grade final paragraph is using similar triangles to solve for slope.
Also, Achieve the Core’s coherence map is deserving of a head nod here:)
Whoever made that thing should blow it up on a wall at the Louvre.