Do You Have Enough Money?

 

You want to use the money you’ve collected in your coin bank to buy the game pictured above.
 

• How do you know if you have enough money in your coin bank to buy the game?
• If there is extra money, how much extra do you have?
• If there is not enough money, how much more do you need?

 

These questions may be useful in helping students down the problem solving path:

• How can we figure out if we have enough money?
• How much money is in the bowl?
• What is a guess that is too low?
• What is a guess that is too high?
• What is your best guess?

 

This problem begins with the goal of determining if you have enough money to purchase the game.  This is where you want to ask students “How can we figure out if we have enough money?”  Students should come up with the idea that we need to find out how much is in the bank.  Once they ask for that information, show them this video:

 

This is a good opportunity to ask students, “How much money is in the bowl?”  You can scaffold that question by asking them for a guess that is too low/high and their best guess.  You want them to articulate that they need to be able to see the money better to make a better guess.  If you have coin manipulatives, feel free to use them at any point in the lesson as a tool to help make the strategies more concrete.  Once they ask to be able to see the money better, show them this video:

 

They should realize that this view is more helpful than viewing the money in the bowl, however the money is difficult to count when it’s disorganized.  We want students to realize that simply picking a coin randomly and adding it to the total will be less efficient than first sorting out the money into groups of the same value (such as piles of pennies, nickles, dimes, quarters, and dollar coins).  This is an important conversation to have as not all students will have familiarity with counting money.  Also, the ability to sort and group money is the foundation for combining like terms later in Algebra where you have to realize that just like 4 quarters + 3 dimes + 5 quarters + 2 dimes = 9 quarters + 5 dimes, 4x + 3y + 5x + 2y = 9x + 5y.

Ask students if they would like to update their guesses.  Once they ask for the coins to be sorted into groups of the same value, show them this video:

 

Sorting the coins into groups should make it easier to count than when it was in one big group, however coins within the groups of the same value are still disorganized making it challenging (or at least inefficient) to count.  Students will be forced to count every coin rather than using repeated addition or multiplication.

Ask students if they would like to update their guesses.  Once students ask for the coins to be organized within each group, show them this video:

 

With the coins finally sorted into organized groups of the same value, students can determine if they have enough money to buy the game.  The image below shows the coins clearly and can be printed out for students:

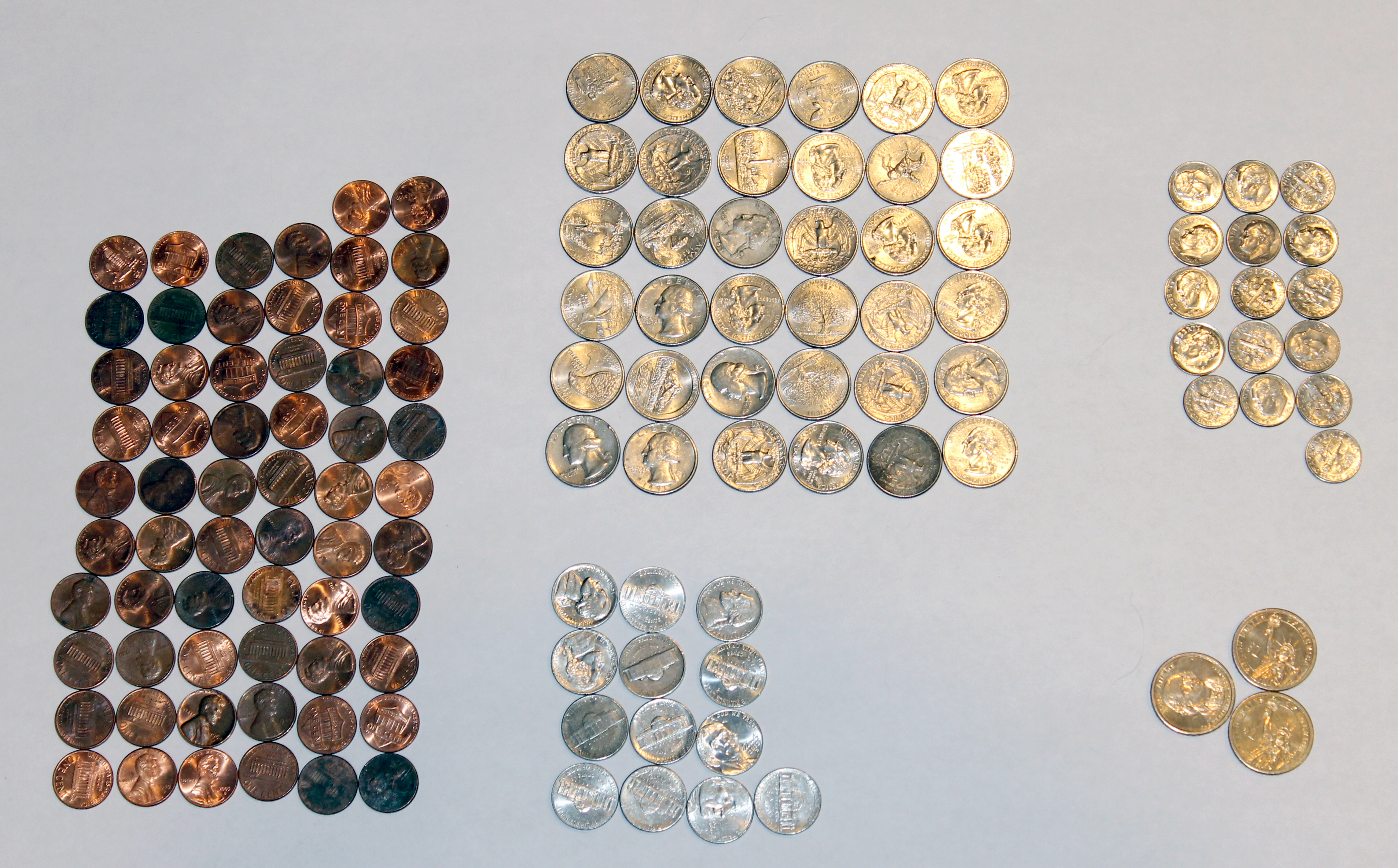
Dan Meyer also made the video below as a more engaging way of displaying the answer.

Students will likely have a variety of strategies for counting how many of each coin there are.  This provides a very rich opportunity for students to share their their mathematical reasoning.  For example with the pennies, students could come up with 62 by:

• 1 + 1 + 1 + … + 1   (adding one plus one 62 times)
• 6 + 6 + 6 + 6 + 6 + 6 + 6 + 6 + 6 + 6 + 2
• 10 + 10 + 10 + 10 + 10 + 10 + 2
• 6 x 10 + 2
• 10 x 6 + 2
• 10 + 10 + 10 + 10 + 11 + 11
• etc.

There are some amazing opportunities for discussion here.  In just one conversation about the strategies students used to count the coins, these Standards for Mathematical Practice can come out:

• Math Practice 1 – Students have to use different different strategies for counting the coins and can change strategies if their first one is not successful.  They should also be able to understand other students’ strategies.
• Math Practice 2 – Students need to be able to take the quantities of coins out of context to add them up, but can students give the numbers back their context?  Can they point out where the the “10”, “6”, “10 x 6”, “2”, etc. is?
• Math Practice 3 – Students need to be able to articulate their reasoning as to how they figured the quantities.  They should also be able to point out flaws in other students’ reasoning.
• Math Practice 4 – Students are applying “the mathematics they know to solve problems arising in everyday life.”  They need to learn what strategies are available and which are more efficient.
• Math Practice 5 – Students are able to use their guesses as estimates for the overall value to help them determine whether their answers are reasonable.
• Math Practice 6 – Students need to be able to specify what quantity they are talking about.  Is 62 the number of dollars, cents, quarters, dimes, nickles, pennies, or something else?  Can it be more than one of them?
• Math Practice 7 – Students should be able to use the coins’ organization as an array that can be used for repeated addition or multiplication rather than counting 62 individual coins.  This sets them up for future properties of mathematics such as the commutative property of multiplication since they can see that 10 groups of 6 (10 x 6) is the same as 6 groups of 10 (6 x 10) so 10 x 6 = 6 x 10.
• Math Practice 8 – Students should use the coins’ patterns to increase efficiency.  For example, instead of adding one sixty-two times, they should switch to adding by 6s or 10s when they see the calculations repeating.

 

In total, there are 62 pennies, 36 quarters, 13 nickles, 16 dimes, and 3 dollar coins for a total of $14.87 (Thanks to the great teachers from Hall County for pointing out that I had previously listed $14.27).  So unfortunately there is not enough money to buy the game (with or without tax and shipping).

As an extension, you can ask students how much more money we would need to be able to buy the game.  Thanks to Andrew Stadel for his time and thoughtful suggestions while I was creating this lesson as well as for the extension questions.
 

• CCSS 2.MD.8 Solve word problems involving dollar bills, quarters, dimes, nickels, and pennies, using $ and ¢ symbols appropriately. Example: If you have 2 dimes and 3 pennies, how many cents do you have?
• CCSS 3.OA.8 Solve two-step word problems using the four operations. Represent these problems using equations with a letter standing for the unknown quantity. Assess the reasonableness of answers using mental computation and estimation strategies including rounding.
• CCSS 3.OA.5 Apply properties of operations as strategies to multiply and divide.
• CCSS 4.MD.2 Use the four operations to solve word problems involving distances, intervals of time, liquid volumes, masses of objects, and money, including problems involving simple fractions or decimals, and problems that require expressing measurements given in a larger unit in terms of a smaller unit. Represent measurement quantities using diagrams such as number line diagrams that feature a measurement scale.

 

• Amazon