- When would both toilets use the same amount of water?
- What information do we need to know?
- When would the dual flush toilet obviously use less water?
- When would the single flush toilet obviously use less water?
- How could the two toilets use about the same amount of water?
- What assumptions are we making?
The information that you need to know (and what may not be easily readable on the image) is the amount of water each toilet uses. The toilets use the unit gpf or gallons (of water used) per flush. The dual flush toilet uses 1.6 gpf for “solids” and 1.0 gpf for “liquids” while the single flush always uses 1.28 gpf.
From my experiences, people approach this problem from one of two ways:
- Some attempt this problem as a series of guess and check ratios. Specifically, they know that the single flush toilet always uses 1.28 gpf. So, they try to find out what ratio of “liquids” to “solids” on the dual flush toilet also gives an average of 1.28 gpf. They might start with 1:1 and realize that averages 1.3 gpf. Then they might try 2:1 and realize that averages 1.2 gpf. From there they narrow down the ratio until they eventually get to 8:7 which has a ratio of exactly 1.28 gpf.
- Others attempt this problem using one of these two systems of equations (which is my ultimate goal for this lesson):
- 1.0*x + 1.6*y = total water usage AND 1.28*x +1.28*y = total water usage
- Where x is the number of “liquid” flushes and y is the number of “solid” flushes
- Set then equal to each other and solve
- x*1.0 + y*1.6 = 1.28 AND x + y = 1
- Where x is the percentage of “liquid” flushes and y is the percentage of “solid” flushes
- The first equation describes the percentage of flushes for “liquids” and “solids” that would average out to 1.28 gpf
- The second equation has those two percentages adding up to 1 (or 100%)
- 1.0*x + 1.6*y = total water usage AND 1.28*x +1.28*y = total water usage
If students get stuck, consider sharing these three scenarios:
- You use the dual flush toilet 100% of the time for “liquids”. So, it uses 1.0 gpf which is less water than the 1.28 gpf single flush toilet.
- You use the dual flush toilet 100% of the time for “solids”. So, it uses 1.6 gpf which is more water than the 1.28 gpf single flush toilet.
- You use the dual flush toilet 50% of the time for “liquids” and 50% of the time for “solids”. So, it averages 1.3 gpf which is more than the 1.28 gpf single flush toilet.
Using this type of reasoning, students should be able to reach the conclusion that the toilets’ water usage would be about the same when the dual flush toilet is used slightly less than 50% of the time for “solids” and slightly more than 50% of the time for “liquids”.
One last note: for a historical perspective, most toilets used to use 3.4 gpf. Today toilets now use 1.6 gpf or even 1.28 gpf. So, both of these toilets would save water compared to older models.
I have also left the prices in the pictures in case you want to also find out when one toilet would pay for itself from the water savings.
- Picture of dual flush toilet

- Picture of single flush toilet

- Low

- Low
- Medium

- High

- High

Where I try to combine @robertkaplinsky ‘s “Which Toilet” problem http://t.co/Q3ry1PwRes with @Desmos https://t.co/lxQAkOssmp #mathchat
— Karl Fisch (@karlfisch) October 24, 2013
- CCSS 8.EE.8c Solve real-world and mathematical problems leading to two linear equations in two variables. For example, given coordinates for two pairs of points, determine whether the line through the first pair of points intersects the line through the second pair.
- CCSS A-CED.3 Represent constraints by equations or inequalities, and by systems of equations and/or inequalities, and interpret solutions as viable or nonviable options in a modeling context. For example, represent inequalities describing nutritional and cost constraints on combinations of different foods.
- CCSS A-REI.11 Explain why the x-coordinates of the points where the graphs of the equations y = f(x) and y = g(x) intersect are the solutions of the equation f(x) = g(x); find the solutions approximately, e.g., using technology to graph the functions, make tables of values, or find successive approximations. Include cases where f(x) and/or g(x) are linear, polynomial, rational, absolute value, exponential, and logarithmic functions.
- CCSS F-BF.1 Write a function that describes a relationship between two quantities.



I am going to hook the task by showing ” It’s a Privilege to Pee” from Urinetown. I have picked out a 4 min video from youtube. A lot of my students don’t have exposure to fine arts. This hook opens up other possibilities for this lesson and later lessons.
I also took some pictures at the local NFL stadium this afternoon. The ladies bathrooms in the 100 level have the flushing options as above. I have pictures of the toilets and the signs. By showing the pictures, it will pull the idea closer to home and make it real. The stadium is about 15 min, without traffic, from the School.
Funny. I’ve never seen or heard of that video. I’ll check it out. Let me know how connecting it to the local stadium goes.
🙂 The kids loved both. Urinetown won a few Tony’s around the time they were born, so none had seen the musical. They were mesmerized by the video and the topic hooked them right away. A lot of good real life math and “life” chatter followed.
They thought I was a little odd for taking pictures of the toilets, but it did bring it close to home. And it brought up other comments like ” Oh yeah they have those at Walmart….”
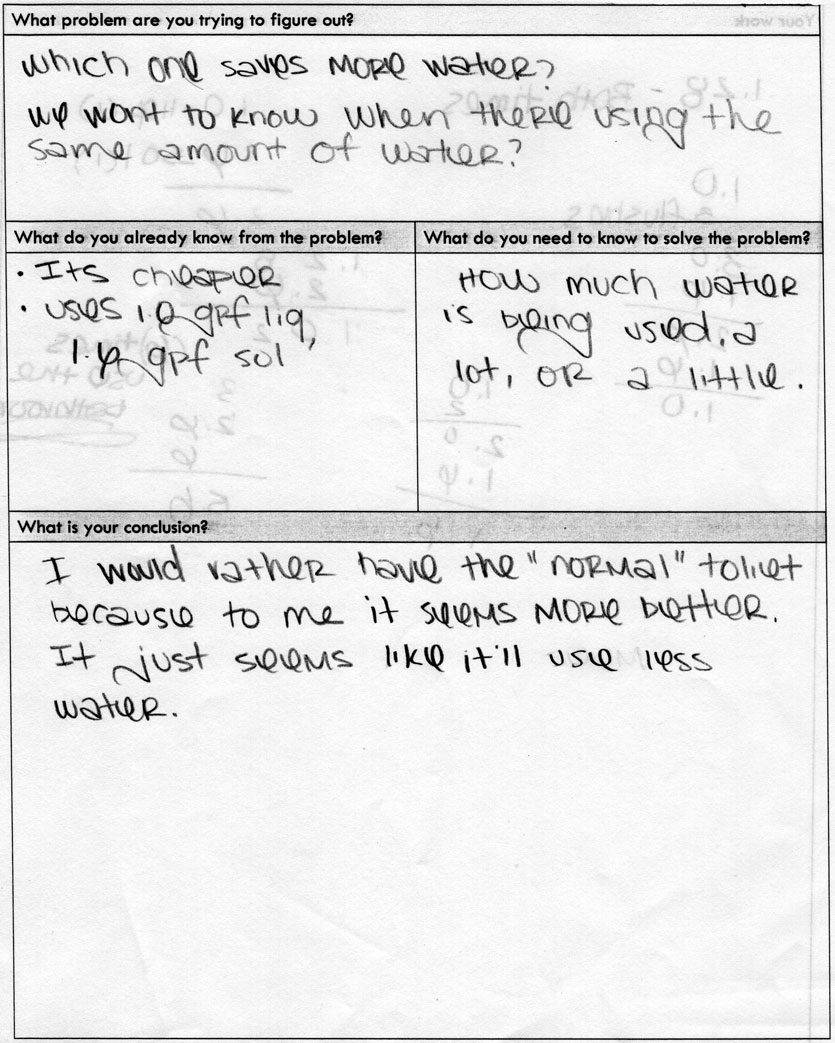
I used the “several attempts” sheet from openmiddle with it. I recreated the sheet from above.
There was a lot of productive struggle and authentic math thinking as they went through the process. It was good stuff.
Basic stats: the class is 70% IEP & 504 and 58% FARMS. (both percents truncated).
Awesome. Glad it went well. FYI, the sheet above is called the Problem Solving Framework and you can download it on the Resources page here: http://robertkaplinsky.com/resources/.
I was a little confused by the questions:
1) “When would the dual flush toilet obviously use less water?”
2) “When would the single flush toilet obviously use less water?”
3) “How could the two toilets use about the same amount of water?”
I wandered around a bit with off-topic answers like “If only one toilet is being flushed, the other uses less water. If neither is being flushed–and neither leaks–then they use the same amount. Or, if I pour .28 gallons of water into the dual-flush toilet bowl, then they would flush the same amount.”
I tried to rephrase the questions to help me focus on the target, and the best I came up with was “How might the pattern of toilet usage over time in your house make one of these toilets a wiser purchase than the other?”
On another note, question (3) above can be solved very quickly by a certain use of weighted averages. In this case, we want to know the weights (corresponding to frequency of that kind of flush) on 1 gal and 1.6 gal that produce a weighted average of 1.28 gal. Since the distances from 1.28 gal to the endpoints (1 gal and 1.6 gal) are .28 gal and .32 gal, respectively, the weights should be in a 32:28 ratio, which is to say 8:7.
This procedure that I’ve just outlined puts me in a pickle. Pro: it’s magically fast. Con: it’s good only for the special case of finding the weights in a weighted average, a.k.a. convex combination, of two things. Pro: that special case sure turns up a lot. Con: it doesn’t *lead* anywhere–nobody (in K-12) builds later content on this idea. Pro: students can learn to execute the procedure long before they see algebra. Con: who’s going to *understand* it, before they chew on some algebra for a while?
As much as I love this method, I don’t really know whom to share it with. I hope you or some of your readers might have an idea about that–and I’d love to be proven wrong about some of those cons.
Well…I have two ideas whom to share it with: first, people who collect shortcuts (hopefully not to the exclusion of other skills); second, people who have learned enough algebra that they are ready to use it as a tool for reflecting on arithmetic. (This puts it in the same category as squaring numbers that end in 5, or multiplying two numbers via the difference of two squares, etc.)
I’m not really good at maths stuff but the way you explained it is so easy for me to understand. Thanks for an amazing guide, Robert!